It's a Wonderful Line: Data Analysis Using Graphs
Conservation Laws - Data Analysis Using Graphs - Histograms - Units or Vectors in Particle Physics
Example 1 - Example 2 - Example 3 - Example 4 - Problems
Few things in science are as daunting as a pile of unreduced data. "Here's a bunch of numbers. Draw a conclusion." Talk about a plunge into the icy cold water! Fortunately, we have graphing as a powerful way to help us dry off, visualize the data and translate it into mathematical relationships.

Example 1: A particle in a magnetic field
We know that if a charged particle like a proton with some momentum p enters a magnetic field, its path will be bent into a circle or a section of a circle. We ought to be able to infer the magnitude of p from the radius of curvature of the path R and from the magnetic field B. But how?

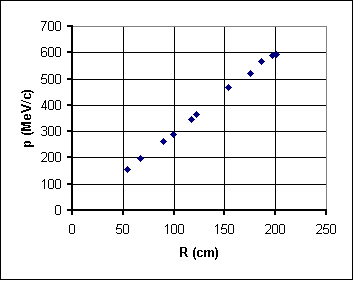
Below we have hypothetical data from an experiment in which particles of various known momenta are injected into a 10 T magnetic field. The data table is to the left. On the right is a graph of that data.

Radius R (cm)
Momentum p (MeV/c)
54
168
67
195
90
279
99
292
117
348
122
368
154
458
175
526
186
563
197
595
201
598
The data points in the graph form a pretty good straight line. It's not perfect—some variation in the data is to be expected, but it is clearly a line which intercepts the axes at the origin. Straight lines take the form y = mx + b but here momentum p in MeV/c takes the place of y and radius R in cm takes the place of x. We already said that the y-intercept, b, is equal to zero. So we get p = mR where m is the slope of the line. Let's draw a line of best fit and find the slope:



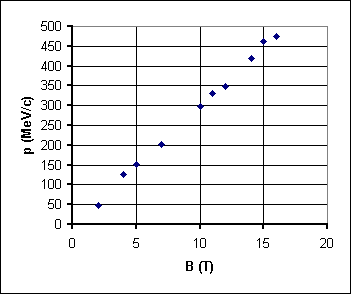
The slope m equals the "rise" divided by the "run." m = 450/150 = 3.0 MeV/c-cm Thus p = (3.0 MeV/c-cm)R Great! We have derived a mathematical relationship from data! (They say every time a mathematical relationship is derived somewhere a physicist gets accelerator time. Or is that only an old story?) However . . . What about B? This data is for a steady B = 10 T. So let's now look at data for momentum p as a function of B, with R held constant:

B (T)
P (MeV/c)
2
50
4
136
5
144
7
201
10
305
11
322
12
354
14
439
15
460
16
486
Clearly, this is also a straight-line relationship. So if p is linear in B and, as we saw previously, also linear in R, then we can say p = kBR where k is some constant. But wait! We know that when B = 10 T p = (3.0 MeV/c-cm)R Then we can say kB = 3.0 MeV/c-cm

k(10 T) = 3.0 MeV/c-cm

k = 0.30 MeV/c-cm-T

and thus p = (0.30 MeV/c-cm-T)BR Using two graphs, we've established a relationship—a sturdy mathematical equation—between p, B, and R. This equation can be found in textbooks and is derivable from the Lorentz force, though our units here are a bit odd outside the world of particle physics.

The key to our success has been using the equation for a straight line. However, not all relationships show a straight-line relationship. What do we do then?

Example 2